This article is not a course in layout. However, it will give you some basic skills needed on the job. You will learn some basic principles that apply to constructing many different shapes.

Swinging an arc (Figure 2) with dividers or compass means keeping one leg fixed and drawing an arc with the other.

ANGLES
Any shape that is made of straight lines has angles, so knowing how to measure angles and how to use them is a basic skill in HVAC work.An angle is the figure formed by the intersection of two straight lines. Figure 3 shows various angles. The most common angle is a square corner. Most of the angles you work with on the job are square corners.
The vertex of an angle (Figure 3) is the point where the two legs (sides) of the angle meet. It is often called the centerpoint. An acute angle is smaller than a square corner. An obtuse angle is larger than a square corner (Figure 3). Notice the symbol in Figure 3 that indicates a square corner (90 degrees) and the symbol for any angle.

Just as a length is measured in inches, angles are measured indegrees. A square corner contains 90 degrees (Figure 3). A 45 degree angle (Figure 3), which is half of a 90 degree angle, is widely used. A 30 degree angle is also common. The length of the legs on the angle has nothing to do with the number of degrees in the angle. Both of the angles in Figure 4 are 45 degree angles.

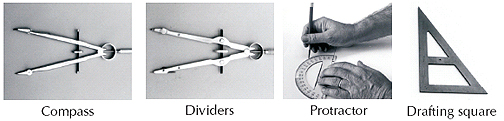
Angles are measured with a protractor. A flat protractor (Figure 1) is used for drafting. A sliding blade protractor (Figure 7) is used in the shop and on the job.

Angles in the HVAC industry are seldom measured to a greater accuracy than whole degrees. However, you should be aware that the degree is divided into 60minutes. The angle 12 degrees, 30 minutes is the same as 12-1/2 degrees.
You are not likely to need to use degrees divided into minutes in work in the HVAC industry. You should know the term in case you run into it.

The most common shapes with straight sides (squares and rectangles) are made with 90 degree angles. It is important to be able to construct 90 degree angles to make these figures accurately.
To develop a 90 degree angle, draw a straight horizontal line and then erect a line that is perpendicular to it. This means that it is straight up and down in relation to the horizontal line. The angle formed on each side is 90 degrees. The procedure is called squaring up a line or erecting a perpendicular. You can do this by using a drafting square to trace a 90 degree angle (Figure 8).


Copying an Angle
Sometimes an angle must be measured on the job in order to duplicate it in the shop or drafting room. There are two common ways to this. You can use a rule to copy an angle (Figure 10):

You can also use a rule and dividers or compass to copy an angle (Figure 11):

Bisecting an Angle
Angles often have to be bisected (divided into two equal parts). Bisecting an angle may be a useful way to create a given angle. For example, to create a 45 degree angle, you can bisect a 90 degree angle. To create a 15 degree angle, you can bisect a 30 degree angle. Use dividers and rule to bisect an angle (Figure 12):

Dividing an Angle in Three Parts
An angle can be divided into three equal parts. This could be done to create a given angle. For example, to create a 30 degree angle, divide a 90 degree angle into three parts. To create a 60 degree angle, use two of the 30 degree angles formed by dividing a 90 degree angle into three parts. Follow this procedure to divide an angle into three parts (Figure 13):
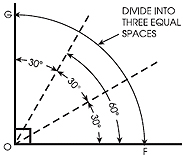
Draw lines from point O through each of the dividing lines on the arc. If the original angle is 90 degrees, then the three angles are each 30 degrees. Two of the divisions make a 60 degrees angle.
The same method can be used to divide an angle into four, five, or any other number of equal parts.
STRAIGHT LINE FIGURES
You may have to sketch ducts, plenums, rooms, buildings, and other structures which are usually made up of straight line figures such as squares and rectangles. You may have to deal with trapezoids, pentagons, and other shapes as well. All of these figures are made of straight lines and angles.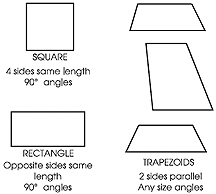
A trapezoid (Figure 14) has two sides that are parallel and two sides that are not parallel. The angles could have any number of degrees. Some rooms, buildings, or windows may be trapezoids. To copy a trapezoid, use a ruler to measure the sides. Copy the angles with the method described above in "Copying an Angle" (or use a protractor).

Pentagon - five sides
Hexagon - six sides
Octagon - eight sides
If the sides are equal, the angles will all be equal. You can copy any such figure by measuring the sides and copying the angle.
CIRCLES
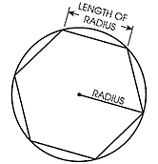
A circle is another basic figure used in the industry. You will often deal with round ducts. Sometimes rooms or buildings are based on circles or parts of circles. The basic parts of the circle are shown in Figure 17: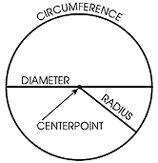
Use a compass to draw a circle. Set a compass to the length of the radius required in order to copy a circle or arc.
Excerpted and reprinted from Math for the Technician by Leo A. Meyer, one of the books in the Indoor Environment Technician's Library series published by LAMA Books.
Publication date: 11/13/2006








